
System Selection Guide
Mirion offers guidance with selections to meet your needs, based on application, software and hardware.
Mirion offers guidance with selections to meet your needs, based on application, software and hardware.
DETECTOR OVERVIEW
The kinds of detectors commonly used can be categorized as:
The choice of a particular detector type for an application depends upon the X-ray or gamma energy range of interest and the application’s resolution and efficiency requirements. Additional considerations include count rate performance, the suitability of the detector for timing experiments, and of course, price.
DETECTOR EFFICIENCY
The efficiency of a detector is a measure of how many pulses occur for a given number of gamma rays. Various kinds of efficiency definitions are in common use for gamma ray detectors:
Clearly, to be useful, the detector must be capable of absorbing a large fraction of the gamma ray energy. This is accomplished by using a detector of suitable size, or by choosing a detector material of suitable high Z. An example of a full-energy peak efficiency curve for a germanium detector is shown in Figure 1.1.

Figure 1.1 - Efficiency Calibration
DETECTOR RESOLUTION
Resolution is a measure of the width (full width half max) of a single energy peak at a specific energy, either expressed in absolute keV (as with Germanium Detectors), or as a percentage of the energy at that point (Sodium Iodide Detectors). Better (lower FWHM value) resolution enables the system to more clearly separate the peaks within a spectrum. Figure 1.2 shows two spectra collected from the same source, one using a sodium iodide (NaI(TI)) detector and one using germanium (HPGe). Even though this is a rather simple spectrum, the peaks presented by the sodium iodide detector are overlapping to some degree, while those from the germanium detector are clearly separated. In a complex spectrum, with peaks numbering in the hundreds, the use of a germanium detector becomes mandatory for analysis.

Figure 1.2
GAS-FILLED DETECTORS
A gas-filled detector is basically a metal chamber filled with gas and containing a positively biased anode wire. A photon passing through the gas produces free electrons and positive ions. The electrons are attracted to the anode, producing an electric pulse.
At low anode voltages, the electrons may recombine with the ions. Recombination may also occur for a high density of ions. At a sufficiently high voltage nearly all electrons are collected, and the detector is known as an ionization chamber. At higher voltages the electrons are accelerated toward the anode at energies high enough to ionize other atoms, thus creating a larger number of electrons. This detector is known as a proportional counter. At higher voltages the electron multiplication is even greater, and the number of electrons collected is independent of the initial ionization. This detector is the Geiger-Mueller counter, in which the large output pulse is the same for all photons. At still higher voltages continuous discharge occurs.
The different voltage regions are indicated schematically in Figure 1.3. The actual voltages can vary widely from one detector to the next, depending upon the detector geometry and the gas type and pressure.
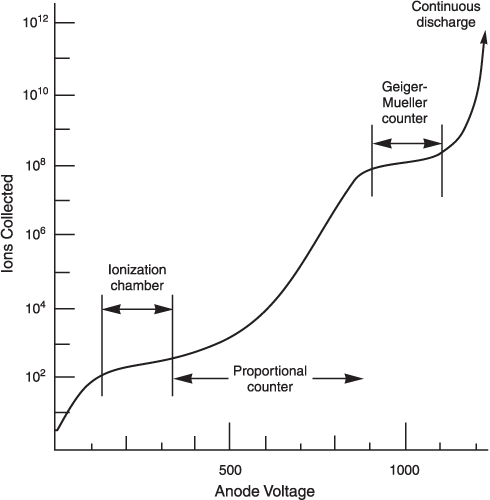
Figure 1.3 - Gas Detector Output vs. Anode Voltage
IONIZATION CHAMBER
The very low signal output for the ionization chamber makes this detector difficult to use for detecting individual gamma rays. It finds use in high radiation fluxes in which the total current produced can be very large. Many radiation monitoring instruments use ionization chambers. Absolute ionization measurements can be made, using an electrometer for recording the output.1
PROPORTIONAL COUNTER
Proportional counters are frequently used for X-ray measurements where moderate energy resolution is required. A spectrum of 57Co is shown in Figure 1.5 in which 14.4 keV gamma rays are wellseparated from the 6.4 keV X rays from iron.
Proportional counters can be purchased in different sizes and shapes, ranging from cylindrical with end or side windows to “pancake” flat cylinders. They may be sealed detectors or operate with gas flow, and may have thin beryllium windows or be windowless. A detector is typically specified in terms of its physical size, effective window size and gas path length, operating voltage range and resolution for the 5.9 keV X ray from a 55Fe source (Mn X ray). Typical resolutions are about 16 to 20% full-width at half maximum (FWHM).
Operating voltages depend upon the fill gas as well as the geometry. For X rays, noble gases are often used, with xenon, krypton, neon and argon common choices. Xenon and krypton are selected for higher energy X rays or to get higher efficiencies, while neon is selected when it is desired to detect low energy X rays in the presence of unwanted higher energy X rays. Sometimes gas mixtures are used, such as P-10 gas, which is a mixture of 90% argon and 10% methane. Gas pressures are typically one atmosphere. The 2006 preamplifier available for proportional counters is shown in Figure 1.4.
Figure 1.4 - Proportional Counter and Preamplifier
GEIGER-MUELLER COUNTER
The Geiger-Mueller counter produces a large voltage pulse that is easily counted without further amplification. No energy measurements are possible since the output pulse height is independent of initial ionization. Geiger-Mueller counters are available in a wide variety of sizes, generally with a thin mica window. The operating voltage is in the plateau region (see Figure 1.3), which can be relatively flat over a range of bias voltage. The plateau is determined by measuring the counting rate as a function of the anode voltage.
The discharge produced by an ionization must be quenched in order for the detector to be returned to a neutral ionization state for the next pulse. This is accomplished by using a fill gas that contains a small amount of halogen in addition to a noble gas. The voltage drop across a large resistor between the anode and bias supply will also serve to quench the discharge since the operating voltage will be reduced below the plateau.
The Geiger-Mueller counter is inactive or “dead” after each pulse until the quenching is complete. This dead time can be hundreds of microseconds long, which limits the counter to low count rate applications.
SCINTILLATION DETECTORS
A gamma ray interacting with a scintillator produces a pulse of light, which is converted to an electric pulse by a photomultiplier tube. The photomultiplier consists of a photocathode, a focusing electrode and 10 or more dynodes that multiply the number of electrons striking them several times each. The anode and dynodes are biased by a chain of resistors typically located in a plug-on tube base assembly. Complete assemblies including scintillator and photomultiplier tube are commercially available from Mirion.
The properties of scintillation material required for good detectors are transparency, availability in large size, and large light output proportional to gamma ray energy. Relatively few materials have good properties for detectors. Thallium activated NaI and CsI crystals are commonly used, as well as a wide variety of plastics. LaBr3 (Ce) crystals are a newer type of scintillation detector material offering better resolution, but otherwise, similar characteristics to NaI detector crystals. NaI is still the dominant material for gamma detection because it provides good gamma ray resolution and is economical. However, plastics have much faster pulse light decay and find use in timing applications, even though they often offer little or no energy resolution.
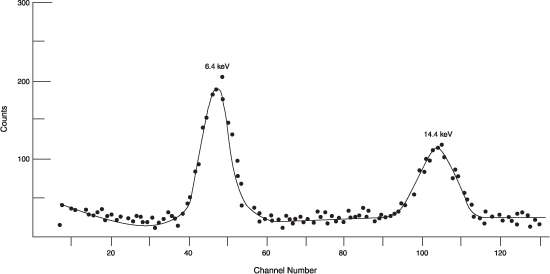
Figure 1.5 - 57Co Spectrum from Counter
NaI(Tl) SCINTILLATION DETECTORS
The high Z of iodine in NaI gives good efficiency for gamma ray detection. A small amount of Tl is added in order to activate the crystal, so that the designation is usually NaI(Tl) for the crystal. The best resolution achievable ranges from 7.5%-8.5% for the 662 keV gamma ray from 137Cs for 3 in. diameter by 3 in. long crystal, and is slightly worse for smaller and larger sizes. Figure 1.7 shows, respectively, the absorption efficiencies of various thicknesses of NaI crystals and the transmission coefficient through the most commonly used entrance windows. Many configurations of NaI detectors are commercially available, ranging from crystals for X-ray measurements in which the detector is relatively thin (to optimize resolution at the expense of efficiency at higher energies), to large crystals with multiple phototubes. Crystals built with a well to allow nearly spherical 4π geometry counting of weak samples are also a widely-used configuration. A typical preamplifier and amplifier combination is shown in Figure 1.6.

Figure 1.6 - NaI(Tl) Detector Electronics
The light decay time constant in NaI is about 0.25 microseconds, and typical charge sensitive preamplifiers translate this into an output pulse rise time of about 0.5 microseconds. For this reason, NaI detectors are not as well-suited as plastic detectors for fast coincidence measurements, where very short resolving times are required. LaBr3 (Ce) detectors have a light decay time constant of 0.03 microseconds making them another possible solution for coincidence measurements.
SEMICONDUCTOR DETECTORS
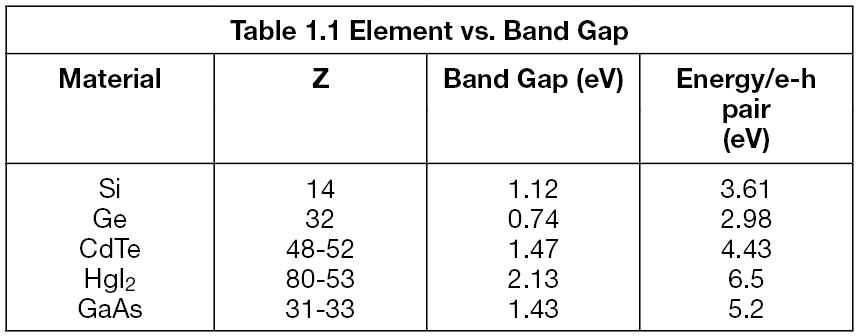
A semiconductor is a material that can act as an insulator or as a conductor. In electronics the term “solid state” is often used interchangeably with semiconductor, but in the detector field the term can obviously be applied to solid scintillators. Therefore, semiconductor is the preferred term for those detectors which are fabricated from either elemental or compound single crystal materials having a band gap in the range of approximately 1 to 5 eV. The group IV elements silicon and germanium are by far the most widely-used semiconductors, although some compound semiconductor materials are finding use in special applications as development work on them continues.
Table 1.1 shows some of the key characteristics of various semiconductors as detector materials:
Semiconductor detectors have a p-i-n diode structure in which the intrinsic (i) region is created by depletion of charge carriers when a reverse bias is applied across the diode. When photons interact within the depletion region, charge carriers (holes and electrons) are freed and are swept to their respective collecting electrode by the electric field. The resultant charge is integrated by a charge sensitive preamplifier and converted to a voltage pulse with an amplitude proportional to the original photon energy.
Since the depletion depth is inversely proportional to net electrical impurity concentration, and since counting efficiency is also dependent on the purity of the material, large volumes of very pure material are needed to ensure high counting efficiency for high energy photons.
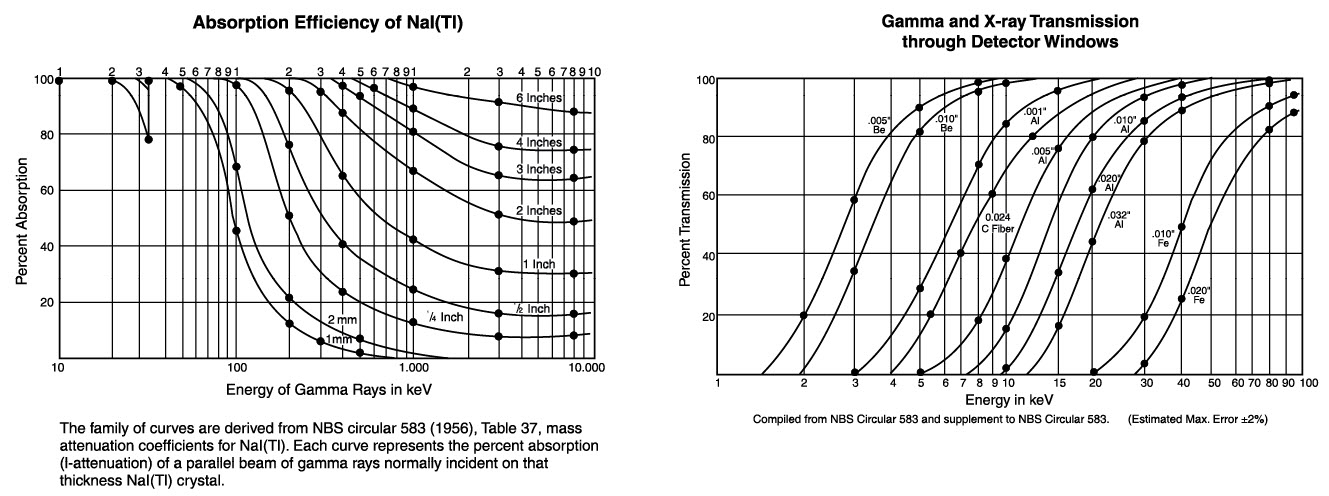
Figure 1.7
Prior to the mid-1970’s the required purity levels of Si and Ge could be achieved only by counter-doping p-type crystals with the n-type impurity, lithium, in a process known as lithium-ion drifting. Although this process is still widely used in the production of Si(Li) X-ray detectors, it is no longer required for germanium detectors since sufficiently pure crystals have been available since 1976.
The band gap figures in Table 1.1 signify the temperature sensitivity of the materials and the practical ways in which these materials can be used as detectors. Just as Ge transistors have much lower maximum operating temperatures than Si devices, so do Ge detectors. As a practical matter both Ge and Si photon detectors must be cooled in order to reduce the thermal charge carrier generation (noise) to an acceptable level. This requirement is quite aside from the lithium precipitation problem which made the old Ge(Li), and to some degree Si(Li) detectors, perishable at room temperature.
The most common medium for detector cooling is liquid nitrogen, however, recent advances in electrical cooling systems have made electrically refrigerated cryostats a viable alternative for many detector applications.
In liquid nitrogen (LN2) cooled detectors, the detector element (and in some cases preamplifier components), are housed in a clean vacuum chamber which is attached to or inserted in a LN2 Dewar. The detector is in thermal contact with the liquid nitrogen which cools it to around 77 °K or –200 °C. At these temperatures, reverse leakage currents are in the range of 10-9 to 10-12 amperes.
In electrically refrigerated detectors, both closed-cycle mixed refrigerant and helium refrigeration systems have been developed to eliminate the need for liquid nitrogen. Besides the obvious advantage of being able to operate where liquid nitrogen is unavailable or supply is uncertain, refrigerated detectors are ideal for applications requiring long-term unattended operation, or applications such as undersea operation, where it is impractical to vent LN2 gas from a conventional cryostat to its surroundings.
A cross-sectional view of a typical liquid nitrogen cryostat is shown in Figure 1.8.
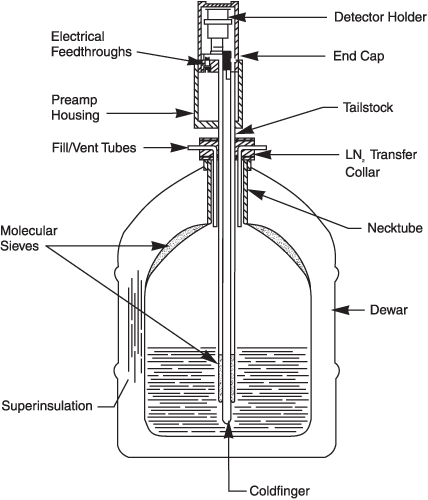
Figure 1.8 - Model 7500SL Vertical Dipstick Cryostat
DETECTOR STRUCTURE
The first semiconductor photon detectors had a simple planar structure similar to their predecessor, the Silicon Surface Barrier (SSB) detector. Soon the grooved planar Si(Li) detector evolved from attempts to reduce leakage currents and thus improve resolution.
The coaxial Ge(Li) detector was developed in order to increase overall detector volume, and thus detection efficiency, while keeping depletion (drift) depths reasonable and minimizing capacitance. Other variations on these structures have come, and some have gone away, but there are several currently in use. These are illustrated in Figure 1.9 with their salient features and approximate energy ranges.

Figure 1.9 - Detector Structures and Energy Ranges
DETECTOR PERFORMANCE
Semiconductor detectors provide greatly improved energy resolution over other types of radiation detectors for many reasons. Fundamentally, the resolution advantage can be attributed to the small amount of energy required to produce a charge carrier and the consequent large “output signal” relative to other detector types for the same incident photon energy. At 3 eV/e-h pair (see Table 1.1) the number of charge carriers produced in Ge is about one and two orders of magnitude higher than in gas and scintillation detectors respectively. The charge multiplication that takes place in proportional counters and in the electron multipliers associated with scintillation detectors, resulting in large output signals, does nothing to improve the fundamental statistics of charge production.
The resultant energy reduction in keV (FWHM) vs. energy for various detector types is illustrated in Table 1.2.

At low energies, detector efficiency is a function of cross-sectional area and window thickness while at high energies total active detector volume more or less determines counting efficiency. Detectors having thin contacts, e.g. Si(Li), Low-Energy Ge and Reverse Electrode Ge detectors, are usually equipped with a Be or composite carbon cryostat window to take full advantage of their intrinsic energy response.
Coaxial Ge detectors are specified in terms of their relative fullenergy peak efficiency compared to that of a 3 in. x 3 in. NaI(Tl) Scintillation detector at a detector to source distance of 25 cm. Detectors of greater than 100% relative efficiency have been fabricated from germanium crystals ranging up to about 75 mm in diameter. About two kg of germanium is required for such a detector.
Curves of detector efficiency vs. energy for various types of Ge detectors can be found in the Detector Product Section of this catalog.
1. A.C. Melissinos, Experiments in Modern Physics, Academic Press, New York (1966), p. 178.
Looking for Services or Support?
We're here to help.